April 2016
(Note: all the previous SPC Knowledge Base in the measurement systems analysis category are listed on the right-hand side. Select this link for information on the SPC for Excel software.)

You take measurements on a process. Maybe your measurements are in increments of 0.01. Is that measurement increment adequate? Is it too small? Why not take measurements in increments of 0.1? The Probable Error helps you determine what size your measurement increment should be.
This month’s publication is designed to help you understand probable error and how it impacts your measurement system. It really defines how precise your measurement system can be – and defines a range of effective increments for your measurement system. Of course, control charts play a role. And Probable Error helps you set your manufacturing specifications – a topic for next month.
In this issue:
- Measurement System Precision
- Measurement System Standard Deviation – What Does It Mean?
- Probable Error Definition
- Using the Probable Error
- Example
- Summary
- Quick Links
Please feel free to leave a comment at the end of the publication. You can also download a pdf copy of this publication at this link.
Measurement System Precision
Most of the time, the precision of a measurement system is reported as an estimated standard deviation. This is easily found by using repeated measurements on the same sample performed by the same operator– and using a control chart to ensure that the results are consistent. Suppose one operator measures the same sample 20 times. The results are given in Table 1.
Table 1: Repeated Measurements of Same Sample
Number | Result | Number | Result |
1 | 99.5 | 11 | 100.0 |
2 | 101.3 | 12 | 99.2 |
3 | 99.2 | 13 | 99.8 |
4 | 99.7 | 14 | 101.2 |
5 | 100.3 | 15 | 99.1 |
6 | 100.3 | 16 | 99.6 |
7 | 100.0 | 17 | 99.3 |
8 | 100.6 | 18 | 99.3 |
9 | 100.8 | 19 | 101.1 |
10 | 101.4 | 20 | 101.0 |
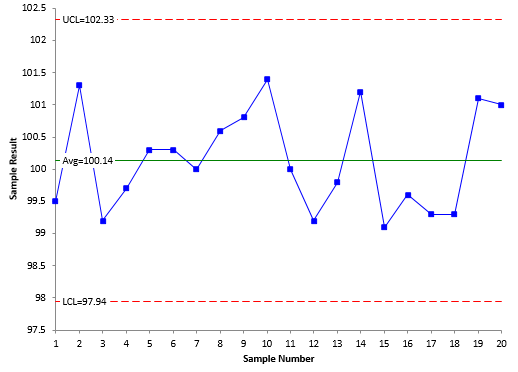
Note that the measurement increment in Table 1 is 0.1. The results in Table 1 were analyzed using an individuals control chart. The X chart is shown in Figure 1.
Figure 1: X Control Chart for Repeated Measurements of Same Sample
Each individual result is plotted on the X control chart. The purpose of this control chart is to determine if the results are consistent. As long as there are no out of control points, the measurement process is consistent with respect to the average. There are no out of control points, so the X control chart is consistent (in statistical control).

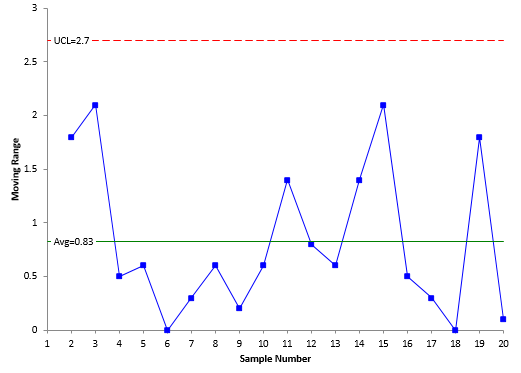
Figure 2: Moving Range Chart for Repeated Measurements of the Same Sample
The other purpose is to estimate the standard deviation of the measurement system. You can do this as long as the moving range control chart is in statistical control. Note that it does not matter if the X control chart is in control or not. The standard deviation for the measurement system (σms) is given by:
σms=R/1.128
The 1.128 value applies only to individuals control charts.
The average range from Figure 2 is 0.83, so σms is given by:
σms=R/1.128 =0.83/1.128=0.74
Measurement System Standard Deviation – What Does It Mean?

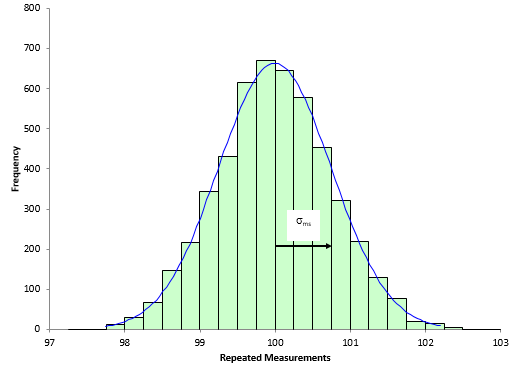
Suppose instead of 20 times, we measured the same sample 5000 times. Wow. Lots of times. To simulate this, 5000 random numbers were generated from a normal distribution population with an average of 100 and a standard deviation of 0.75. The resulting histogram is shown in Figure 3.
Figure 3: Repeated Measurements Histogram
A normal curve has been superimposed on the histogram. So, what does σms mean on this chart? The arrow on the figure depicts the distance from the average (100) to 1 standard deviation above the average (100.75). For a normal distribution, the % of results that fall between the average and 1 standard deviation above the average is about 34%. This means that 34% of the repeated measurements fall between 100 and 100.75. Likewise, about 34% of the repeated measurements fall between the average and one standard deviation below the average (99.25).
The following is true for a normal distribution:
- About 68% of the values fall between -1 and +1 standard deviation from the average
- About 95% of the values fall between -2 and +2 standard deviations from the average
- About 99.97% of the value fall between -3 and +3 standard deviations from the average
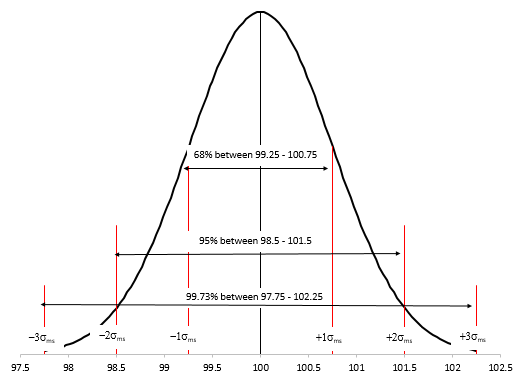
If the “true” average of the sample is 100 and the “true” standard deviation is 0.75, then 68% of the time the repeated measurement will be between 99.25 and 100.75. 95% of the time the repeated measurement will be between 98.50 and 101.50. And 99.97% of the time, the repeated measurement will be between 97.75 and 102.25. This is shown in Figure 4.
Figure 4: Repeated Measurements Normal Distribution
Probable Error Definition

The Probable Error (PE) is defined as the following:
PE = 0.675σms
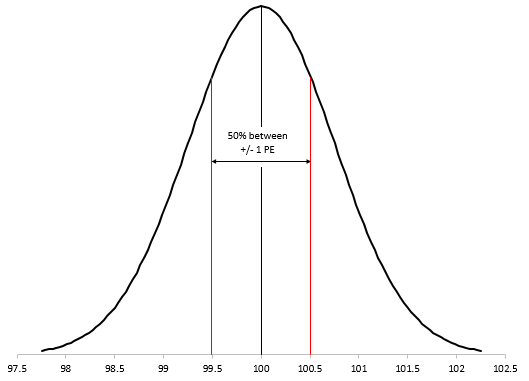
What is special about the value of PE? The PE defines the middle 50% of the normal distribution as shown in Figure 5.
Figure 5: Probable Error
This means that half of the repeated measurements should fall between the average and ± PE as shown below:
X ± 1 PE
X ± 0.675σms
100 ± 0.675(0.75)
100 ± 0.50625
Thus, 50% of the repeated measurements lie between 99.494 and 100.506.
Using the Probable Error
What does this mean for your measurement process? Obviously, it means that 50% of the time the result will within one PE of the average – and 50% of the time it will be outside 1 PE. OK, so what? This actually places limits on the resolution of your measurement system – and the PE can be used to help determine the measurement increment. In Dr. Wheeler’s words:
“No measurement should ever be interpreted as being more precise than plus or minus one Probable Error since your measurement will err by this amount or more at least half the time.”
You can use the PE to determine how many digits you should record your test results at. The goal is to have the measurement increment about the same size of the PE. Dr. Wheeler gives the following guidelines for determining a range of effective measurement increments:
Smallest Effective Measurement Increment = 0.2PE
Largest Effective Measurement Increment = 2PE
Look at the data in Table 1. The measurement increment is 0.1. The PE from above is 0.50625. Thus:
Smallest Effective Measurement Increment = 0.2PE = .2(.50625) = .10125
Largest Effective Measurement Increment = 2PE = 2(.50625) = 1.0125

Example
Ten parts are pulled from a process and measured three times each by one operator. The data are shown in Table 2.
Table 2: Product Data
Subgroup | X1 | X2 | X3 |
1 | 4.57 | 4.39 | 6.25 |
2 | 3.72 | 3.44 | 2.37 |
3 | 3.67 | 3.79 | 3.88 |
4 | 4.23 | 4.12 | 4.47 |
5 | 5.85 | 5.75 | 5.55 |
6 | 3.04 | 3.32 | 3.54 |
7 | 4.52 | 4.65 | 5.68 |
8 | 6.07 | 4.7 | 5.15 |
9 | 3.98 | 3.51 | 4.1 |
10 | 2.31 | 2.83 | 2.58 |
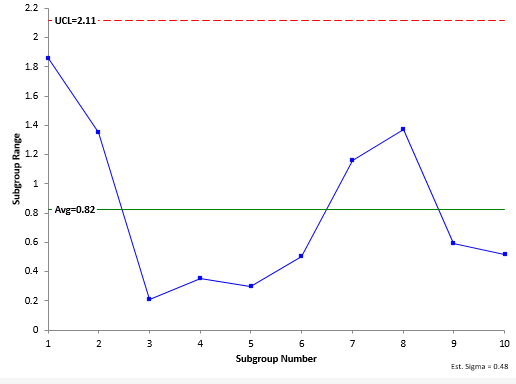
Note that the measurement increment is 0.01. These data can be analyzed using an X-R chart. Here we will only be concerned with the R chart. The R chart is shown in Figure 6.
Figure 6: R Chart for Product Data
The range chart is measuring the variation in the measurement system since the within subgroup variation represents measurements on the same part. It is in statistical control. Thus, we can estimate the measurement system standard deviation as follows:
σms=R/d2 =0.82/1.693=0.48
d2 is a control chart constant that depends on subgroup size (n = 3 in this example). The Probable Error is then given by:
PE = 0.675(σms) = 0.675(0.48) = 0.324
Note that the PE is equal to 32 times the measurement increment. The measurement increment is too small. The range of effective measurement increments is from 0.2PE to 2PE or 0.0648 to 0.648. Thus, the 0.01 digit is not meaningful. The measurement increment should be increased to 0.1
Summary

If two measurements of the same sample differ by less than the probable error, is the difference meaningful? Or are both measurements effectively the same?
Both measurements are effectively the same in my opinion. The difference is not meaningful.
Thanks alot ur work really have fone a lot for me
You are welcome! Thanks for letting me know.!
please tell me how to obtain the constant in the probable error law 0.675
That is the value that defines where 50% of the values lie for a normal distribution. Within +- 0.675 standard deviation of the mean. See figure 5.
Thank you for these really clear explanations. In order to get off to a right start though I am wondering, would the Probable Error in a single measurement eg. of time to the nearest whole minute be:-+/-0.5 minutes,or +/-0.25 minutes (50% or the range) or +/-0.34 minutes (1 Normal SD) ,bearing in mind that each time within the +/- 0.5 minutes range is equally likely for a single measurement? Understanding that Probable Errors add in quadrature, the Probable Error in a length of time between two such measurements would then be:-+/- Squrt (0.52 + 0.52) = +/- 0.707 or +/- Squrt (0.252 +0.252 ) = +/- 0.35or +/- Squrt (0.342 +0.342 ) = +/- 0.48 ?
I am a little confused by what you are asking. The measurement system error is deteremine by repeated measurements on the same part. Call that sigma. Then the PE is 0.675(sigma). 50% of the measurements fall within +/- PE of the average. You use the PE to determine if your measurement increment is valid – as described above.
Where does the 0.2PE and 2PE come from? How to interpret it? What's the impact of your measuring result if this is not followed, either smaller than 0.2 PE or larger than 2PE? Thanks, Taft
Dr. Wheeler provides a good explanation of this at this link:
https://www.qualitydigest.com/inside/statistics-column/last-digit-really-significant-020518.html
I dont understand how to find σms
Hello, you calculate it from the average moving range.
σms= R/1.128 =0.83/1.128=0.74
The moving range is the range between consecutive points. This is an individuals chart. Please see this link for how to calculate the average moving range:
https://www.spcforexcel.com/knowledge/variable-control-charts/individuals-control-charts
Many thanks for the explanation as always!I dont know if this is related question, but is the probable error the same as error bars plotted on a graph?My second question would be in regards to the Standard Deviation, the example above shows a normal distribution. Does the same principle apply for a non normal distribution?Many thanks
YOu are welcome. I don't think it is the probably error, probably just one standard deviation. I don't believe the same principle applies to non-normal distributions but I will have to check that.